
If the sample statistic is too many standard deviations (two) from proportion stated in the null hypothesis, we reject the null hypothesis. hypothesis testing using the classical approach hypothesis testing using the classical approach: z-score A measure of how many standard deviations you are away from the norm (average or mean). three approaches to hypothesis testing Three Approaches to Hypothesis Testing:ģ. In other words: The results basically reveal a flaw in the null hypothesis, because if the null hypothesis were true the observed results would be unlikely.

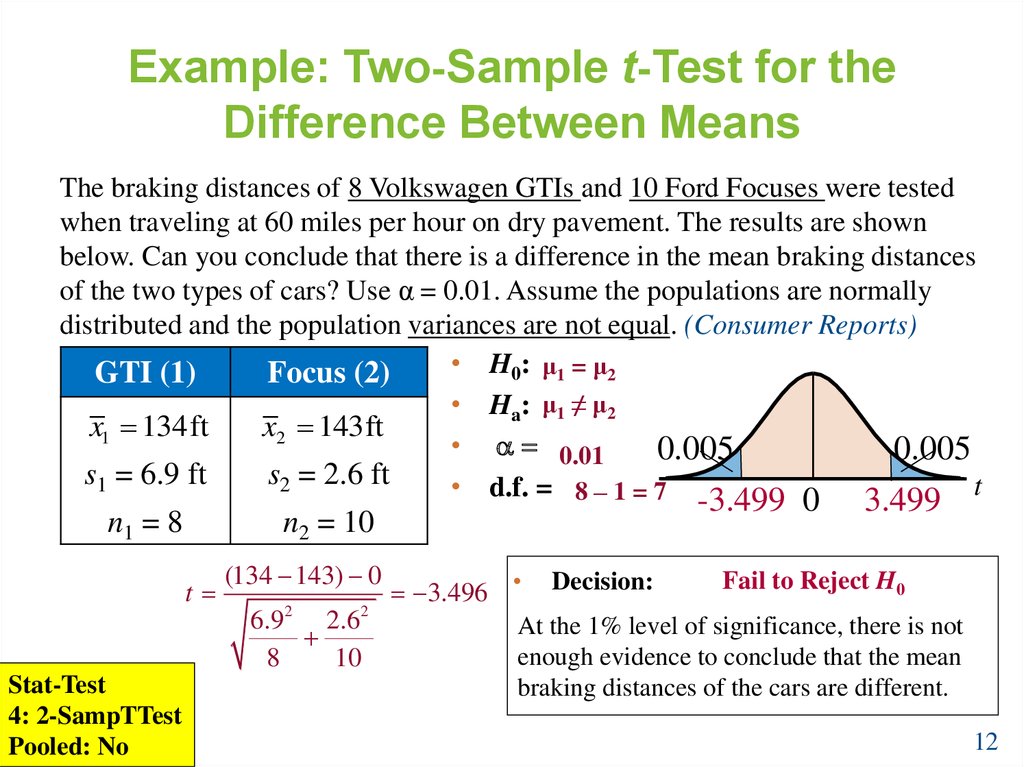
TERMS IN THIS SET (12) statistically significant When observed results are unlikely under the assumption that the null hypothesis is true, we say the results are _ _ and we reject the null hypothesis. usually the dependent or responsible variable is on the y (vertical axis) while the independent variable or predictor variable is plotted on the x (horizontal axis) hidden variables be aware of hidden variables, which may be responsible for patterns we see when graphing or examining relationships between two data sets +61 more terms We should always carefully investigate an outlier before deciding whether to leave it as is, change its value to the correct value, or remove it scatter plot we use scatterplots to visualize the relationship between 2 variablesĬan reveal relationships between two variablesĪlthough there may be a relationship between two variables, we cannot conclude that one variable causes the other - correlation does not imply causation bin range = the number you decided to label each bin outlier a value that falls far from the rest of the data greater than 66 and less than or equal to 68) bins = a range of possible numbers that run up to a number and include that number (e.g. Moreover, it also assumed that the observations are independent and identically distributed.TERMS IN THIS SET (65) histogram we use histograms to help us visualize a variables distributionĪ histograms x-axis represents bins corresponding to ranges of dataĪ histograms y axis indicates the frequency of observations falling into each bin The Student's t-test and the z-test are parametric testsīoth the Student's t-test and the z-test are said to be parametric as their use requires the assumption that the samples are distributed normally. Use the z-test when the true variance σ² of the population is known. Use the Student's t-test when the true variance of the population from which the sample has been extracted is not known the variance of sample s² is used as variance estimator. Two parametric tests are possible but they should be used on certain conditions: The Student's t-test
When to use the Student's t-test or the z-test This tool is used to compare the average of a sample represented by µ with a reference value. XLSTAT - One sample t and z tests Principle of the one-sample t- and z-tests


 0 kommentar(er)
0 kommentar(er)
